Euclid’s Proposition 1:6
The sixth proposition of Book 1 of Euclid’s Elements is the converse of the first part of Proposition 1:5 (If a given triangle is an isosceles triangle, then the two angles at its base are equal to one another). Proposition 1:6 states that if the two angles at the base of a given triangle are equal to one another, then the triangle is an isosceles triangle (in the modern sense of the word):
| Greek | English |
|---|---|
| ̓Εὰν τριγώνου αἱ δύο γωνίαι ἴσαι ἀλλήλαις ὦσιν, καὶ αἱ ὑπὸ τὰς ἴσας γωνίας ὑποτείνουσαι πλευραὶ ἴσαι ἀλλήλαιςἔσονται. | If a triangle has two angles equal to one another then the sides subtending the equal angles will also be equal to one another. |
Euclid does not actually use the word isosceles (ἰσοσκελῶν) in this theorem because he has already defined an isosceles triangle as one in which two and only two sides are equal in length (Definition 1:20). Proposition 1:6, however, admits of the possibility that the given triangle is equilateral, with all three sides equal to one another. In modern geometry equilateral triangles are generally regarded as special cases of isosceles triangles.
In general, the converse of a proposition of the form “If P, then Q” is the proposition “If Q, then P.” When both a proposition and its converse are valid, Euclid tends to prove the converse soon after the proposition, a practice that has continued to this day. ―Joyce
Sometimes the converse of a proposition follows logically from that proposition―a logical operation called conversion. If this is not the case, the converse must be proved independently of the direct proposition.
Euclid proves this theorem by a form of proof by contradiction known as reductio ad absurdum―Latin for reduction to absurdity. Given a triangle in which the angles at the base are equal to one another, Euclid assumes that the two subtending sides are not equal in length. He then proceeds to show how this assumption leads to a contradiction, thus demonstrating that the assumption must be false. In addition to some Definitions, Euclid’s proof requires Postulate 1, Propositions 1:3 and 1:4, and Common Notion 5. It also requires a Common Notion that is not in the Elements. This missing axiom is called the Law of Trichotomy for Lines. Curiously, this Common Notion is not even found in those editions of the Elements that add several extra Common Notions to Euclid’s original five:
Postulate 1 Let it have been postulated to draw a straight-line from any point to any point.
Proposition 1:3 For two given unequal straight-lines, to cut off from the greater a straight-line equal to the lesser.
Proposition 1:4 The Side-Angle-Side Congruence Theorem for Triangles.
Common Notion 5 And the whole [is] greater than the part.
Law of Trichotomy for Lines If AB does not equal AC, then one of them is greater than the other: either AB > AC or AC > AB.
In his translation of the Elements Richard Fitzpatrick also requires another axiom:
Here, use is made of the previously unmentioned common notion that if two quantities are not unequal then they must be equal. ―Fitzpatrick 13
In modern terminology, Euclid’s proof of Proposition 1:6 takes the following form:
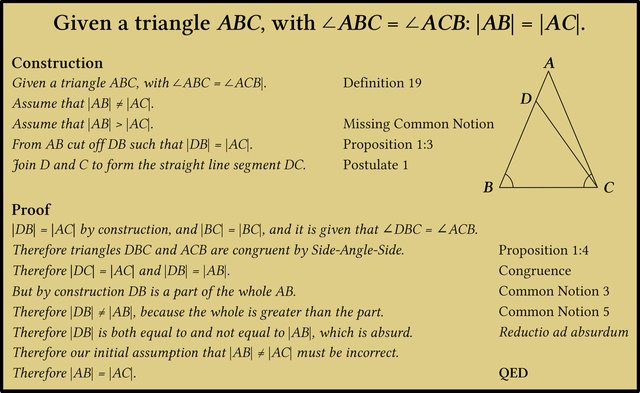
Euclid actually takes triangle DBC as the part and triangle ACB as the whole. Having shown that these triangles are congruent, it follows that the part is equal to the whole, which contradicts Common Notion 5. But strictly speaking, he has only shown that DB is part of AB, so I have altered his proof. Another of Euclid’s translators, Thomas Heath, also raises this point:
Euclid assumes that, because D is between A and B, the triangle DBC is less than the triangle ABC. Some postulate is necessary to justify this tacit assumption; ―Heath 256
Proposition 1:6 is not used elsewhere in Book 1 of the Elements, but it is used in Books 2, 3, 4, 6, and 13.
And that’s a good place to stop.
References
- Ernst Ferdinand August, Euclidis Elementa, Part 1, Theodor Trautwein, Berlin (1826)
- Henry Billingsley (translator), The Elements of Geometrie of the Most Auncient Philosopher Evclide of Megara, John Day, London (1582)
- Oliver Byrne, The First Six Books of The Elements of Euclid in Which Coloured Diagrams and Symbols Are Used Instead of Letters for the Greater Ease of Learners, William Pickering, London (1847)
- Richard Fitzpatrick (translator), Euclid’s Elements of Geometry, University of Texas at Austin, Austin, TX (2008)
- Thomas Little Heath (translator & editor), The Thirteen Books of Euclid’s Elements, Second Edition, Volume 1, Dover Publications, New York (1956)
- Johan Ludvig Heiberg, Heinrich Menge, Euclidis Elementa edidit et Latine interpretatus est I. L. Heiberg, Volumes 1-5, B G Teubner Verlag, Leipzig (1883-1888)
- Henry George Liddell, Robert Scott, A Greek-English Lexicon, Eighth Edition, American Book Company, New York (1901)
- Glenn Raymond Morrow (translator), Proclus: A Commentary on the First Book of Euclid’s Elements, Princeton University Press, Princeton, NJ (1970)
- François Peyrard, Les Œuvres d’Euclide, en Grec, en Latin et en Français, Volumes 1-3, Charles-Frobert Patris, Paris (1814, 1816, 1818)
- Thomas Taylor, The Philosophical and Mathematical Commentaries of Proclus on the First Book of Euclid’s Elements, Volume 1, Volume 2, London (1792)
Online Resources
