Introduction to relativity: events and space-time
Events and space-time
This is the first entry in what hopefully will become a series about relativity, both special and general. I intend to write some articles that will explain relativity in a way understandable also by people with minimal mathematical background. What becomes of it - we'll see ;)
This entry will be quite basic, but I think that some problems will nevertheless be quite interesting. We'll be talking about what is the space-time, events, and we will show where the theory of relativity comes from. So, let's go :)
The notion of space-time is briefly mentioned at school, but usually the profound consequences of combining space and time into a single entity aren't explained too much. To understand this, one must first go a bit deeper into the details of this idea.
The events
Let's start with the basics. Imagine that you want to meet a friend. What do you need to decide? The answer is rather simple:
- where you want to meet
- when you want to meet
The "where" part is usually a few words - this or that restaurant, a movie theater, someone's house, whatever. If, for some reason, you wanted to meet somewhere in the middle of the Himalayas, it will probably be simpler to say two numbers: the latitude and longitude. What, though, if the meeting isn't going to be on the surface of the Earth, but in an underground bunker, or the opposite - on some floating platform? You need a third number, like the altitude above the sea level. Those three numbers - the coordinates - will do the trick: you can meet anywhere on Earth, under or above its surface.
Such a set of coordinates actually lets you denote an arbitrary point in the whole Universe (some of them would just be very high above the Earth's surface), but it's a rather incovenient system, because the Earth constantly moves. Nevertheless, whatever system we come up with, three numbers will always be enough to uniquely identify a point - which stems from the fact that we live in a 3-dimensional space.
To summarise: "where" requires 3 numbers. How about "when"?
We have the calendar and clocks for that, but the description of a moment in time can be reduced into just one number - programmers frequently use one of the ways called a "Unix timestamp". The astronomers have their own system and they use the Julian day (sometimes modified). Anyway, what's important is that time can be described by just one number - we only have one temporal dimension.
These four numbers - three describing a place and one describing the time - are the so called space-time coordinates. A point described by a given set of coordinates, like 52°N, 21°E, 75 m asl, 2000-01-01 12:00 (there are more than four numbers here - but as mentioned before, they can be reduced to four, but it would be less readable) is an event. The space-time itself is then nothing else than the set of all events - in this sense the events are points in space-time. Because we need four numbers to denote a point, this means that the space-time is 4-dimensional.
Okay, we discovered that any event can be described by just four numbers, which leads to a 4-dimensional space-time, but what is so revolutionary about this? Coordinate systems were invented a few hundred years ago, time and space are also known for a long time, and yet nobody before Einstein was particularly interested in the notion of space-time. It's frankly no wonder - if we put space and time together into a single thing, it doesn't mean that we suddenly stop having space and time... right...?
The space-time
Spoiler: wrong. Such a unification of space and time has profound consequences, as we will soon see. But let's not rush.
First, a technical note. Since I'll want to illustrate the text with some drawings, and drawing in 4 dimensions is hard (if you don't agree, please contact me, I'll gladly learn ;) ), we will assume for simplicity that space is just one-dimensional - that it is a line. It won't make a difference for the important things, but it will let us clearly represent everything on a flat screen.
That being said - let us take a look at some John Smith's day.
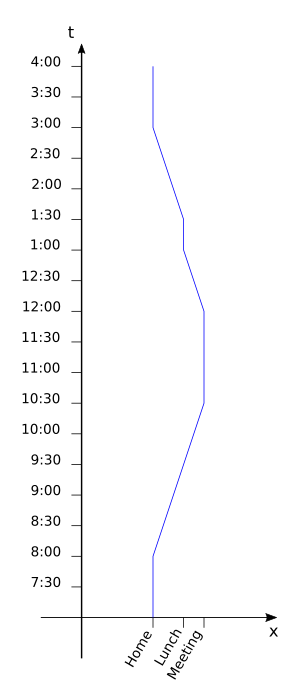
Mr John and the world line
Let's imagine that the hero of our story, John Smith, arranged to meet a friend somewhere he will take 2.5 hours to reach. John left his house at 8 in the morning and got to the meeting at 10:30. He spent an hour and a half with his friend, and then he went travelling home. He got hungry, though, so he stopped for lunch. Eating took him half an hour, after which he went back on the road and reached his house at 3pm.
In the picture to the right we have a diagram showing how John was moving with respect to the Earth. In the beginning, between 7 and 8 in the morning, he was home. Since he wasn't moving then, this part of John's line is vertical - every point on it has the same spatial coordinate, only the time coordinate is changing.
At 8 in the morning John got in his car, or any other means of transportation, and hit the road. As time went by, John kept finding himself in different places (different x coordinates), which causes the line showing this part of the day to be oblique. What's more, the angle of this obliquity (to the vertical direction) corresponds to John's velocity - more oblique line would imply that John covers more distance in the same time, hence he is travelling faster. Here our hero moves with a constant velocity right until the moment of the meeting.
During the meeting John is again at rest, which again results in a vertical line until 12:00, when he starts going back. Then his line becomes oblique again, but to the other side, since he is now moving in the opposite direction. The lunch stop is one more vertical line (no movement again), then one more oblique one for the rest of the travel, until finally John gets home, sits in his chair and his line becomes vertical again.
Such a line, representing what happens to an object as time flows, is called a world line of the object. A world line is generally some curve in the space-time, and every point on it is an event, which the object "experienced".
Towards relativity
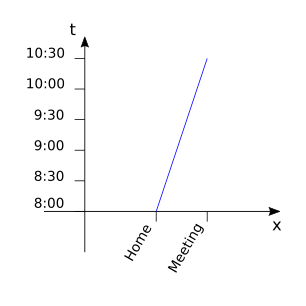
We now know what a world line is and what a space-time diagram looks like, so let's move on. Let's now focus on the part of the day during which John travelled from his house to the meeting (picture to the left).
As we've already said, this part of John's world line is oblique, which means that John was moving. It is also straight, which means that John's velocity wasn't changing - he wasn't accelerating (the slope angle is constant, and as we already mentioned, it corresponds to the velocity). If John was accelerating, the line would be curved a bit upwards (the angle would grow with time), and if he was decelerating, it would be curved the other way - down (shrinking angle).
We have said that John was moving, but there is no objective movement! I used a shortcut here - he was moving with respect to the Earth. With respect to himself, for example, he obviously wasn't - so what would this part of the diagram look like from his point of view?
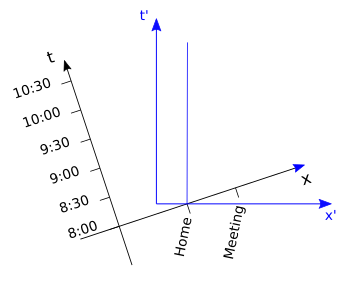
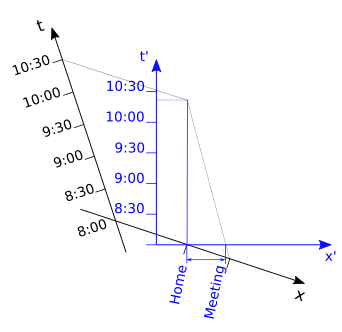
And now the importance of the idea of space-time shows. In John's frame of reference his line must be vertical, but the space-time is still the same. So, in order to see from his point of view, we will rotate our diagram - so that John's line becomes vertical. The result is visible on the right.
The full consequences of this step aren't yet visible, but there are already some strange effects. Since we assume that the relativity principle holds, and it says that all frames of reference are equal, we have to draw the time axis vertically on John's diagram, too. The Earth's time axis shouldn't be anywhere else than where it was, though, so it has to be oblique. Earth's time (t) and John's time (t') are non-parallel!
Let's stress this again: by combining time and space into space-time and drawing a simple picture, we immediately concluded that time is relative and depends on the observer. Immediately! There are no big steps here, you just make a drawing and rotate it! This is the power of the idea of space-time.

For now we just drew the coordinate axes on John's diagram, let's now add ticks to them (well, to the time axis, at least). How dense should the ticks be? Simple - just as dense as on the Earth's time axis, because why should it be different? It makes no difference to a clock if it moves or not - it should just count seconds / minutes / hours along its time axis just the same. You can see ticks drawn this way in the picture on the right.
John left his home at 8:00 and there is no reason for his clock to show different time then. We start at 8:00, then, and we count... But what is happening? At 10:30 John still hasn't reached his destination. Since his world line goes through space-time at an angle, it is a bit longer than the 8:00-10:30 interval on the Earth's time axis. This causes John's clock to show a time later than 10:30 when he gets to the meeting. Weird! (The readers who know something about relativity probably notice that something is off here - we will come back to this.)
Another conclusion can be drawn - different observers can see different time between the same events. We just discovered time dilation!
But this is still not everything. Let's take a look at the distance between John's house and the place of the meeting on the next diagram.
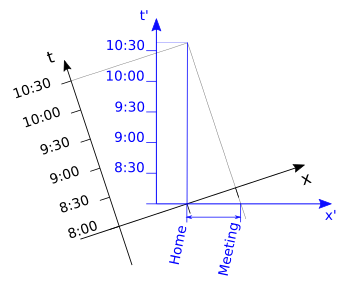
Let's see. The place of the meeting doesn't move with regards to the Earth - so its world line has to be parallel to the Earth's time axis (t). It also has to pass through the appropriate point on the space axis. This is the dotted line on the diagram.
However, John's space isn't actually parallel to Earth's space, just like his time. This causes John's space axis to intersect the meeting place's world line a bit lower. What's more, as a result the interval between John's house and the meeting place on John's space axis is longer than the analogous interval on the Earth's space axis. John thinks he is further from the meeting's place when he moves faster!
There's even more! At 8:00, when John leaves his house, it is before 8:00 at the place of the meeting, according to him! It will be 8:00 there only at the event on the Earth's x axis (it's the place of Earth's 8:00 in the picture), but it will be already later for John! This means that we also discovered the relativity of simultaneity!
We got practically all major consequences of the theory of relativity from a simple diagram. Unfortunately, I had to cheat in a few places and it is now time to set the record straight.
Actual relativity
I already cheated in the third diagram, when we switched our point of view to that of John. I said then that it's enough to rotate the picture and everything will be OK. It would be awesome if that was true, but unfortunately, the reality is more complicated than that. Transformations between frames of reference aren't actually rotations, but rather so called Lorentz transformations.
A more appropriate picture is shown on the right.
The Lorentz transformation is actually very similar to a rotation. Just as rotations move the points along circles, the Lorentz transformations move them along hyperbolas. The space axis gets turned in the opposite direction than the time axis, which results in an unchanging line appearing between them - it corresponds to movement with the speed of light. You can read some more about similarities between hyperbolas and circles here.
What is more important for us here is that some parts of Lorentz transformations work the opposite way to rotations. In the rotate picture it took more than 2.5 hours for John to get to his destination and he had a longer distance to cover. In reality, less time would pass for him (time flows slower for moving observers) and he will have less distance to cover (hence: Lorentz contraction).
Of course, in real life the differences are unnoticeable. This is because if I wanted to be precise in the diagrams, every second on the time axis would have to correspond to about 300 000 km on the space axis - or 1 light-second. If that was the case, John in these diagrams would have traveled light-hours, which is billions of kilometers, in just a few hours - unachievable for a typical person ;) So in fact, world lines for all people are indistinguishable from vertical - but this is not the case for e.g. GPS satellites, which have to take relativistic effects into account.
Regardless of those issues, I think that a look at a sheet-of-paper-space-time, in which rotation is the appropriate transformation, is really helpful in becoming familiar with the ideas of relativity, which are counter-intuitive for many people. Seeing that treating space-time as a whole leads to all sorts of relativistic effects was an enlightening moment for me personally, and I hope that I managed to convey at least a part of this enlightenment here ;)
Summary
As a final note - dear readers, don't hesitate to post questions in the comments. I'll be trying to answer as well as I can. I consider relativity a fascinating topic and I really like to share this fascination :)
This is (hopefully) just a first article in the series - I currently don't know what I'll be describing in the next ones, but we will make small steps towards a description of black holes and fun facts related to them. Stay tuned! :)
(This article has also been posted on my blog.)
References
A. Szymacha Introducing special relativity, IFT 7/92, April 1992 (pdf)
Very nicely explained.
Thanks! :)
This is a very nice way to introduce special relativity. Do you lecture this to students, somehow?
Thanks! No, I'm not a teacher - I did major in physics and I have been tutoring some people, but I've decided to become a software developer and I'm working in this field. I actually like explaining things to people and I've been thinking of becoming a teacher - but let's just say that it's usually not the best career choice in Poland, where I come from ;)
From colleagues working there, the salaries are indeed not that great (although it is apparently easy to get a job). I am looking forward to read the next items in this series :)
Congratulations @ebvalaim! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPnice
Congratulations @ebvalaim! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP