Physics Nobel Prize 2017 - A short introduction to Gravitational Waves
Hey guys, nice to see you 😉
As you are propably aware of, this week all of the winners of the prestigious Nobel Prize are beeing announced.
And with respect to physics the honor goes to Rainer Weiss, Kip Thorne and Barry Barish for the detection of "Gravitational Waves" - a prediction of Albert Einstein's theory of relativity in 1916 and subject of speculation before under Heaviside and Poincaré.
But what is a gravitational wave? I would like to provide a short explanation from the perspective of cosmology:
What are we studying anyway?
To comprehend a gravitational wave one must first understand the physical foundation of our cosmos. To our current knowledge, the laws of nature governing our universe and the behaviour of spacetime are most accurately characterized by Einstein's Theory of General Relativity.
It is the theory which accurately explains various celestial phenomena both qualitatively and quantitatively such as:
- Bending of light trajectories by black holes and massive objects ("Gravity lensing")
- Time dilation in the gravitational field
- Perihelion precession (elliptical orbits e.g. of planets are not static but rotate themselves)
- Gravitational redshift
- etc.
Gravitational Lensing of colliding black holes, LIGO, Simulating eXtreme Spacetimes under CC BY-SA 4.0
The main essence of general relativity observation that we do not live in a 3-dimensional space that evolves with time, but rather in a 4-dimensional spacetime in which time is just one additional (albeit discerned and special) dimension of our world.
This spacetime is mathematically described in term of a peudo-Riemannian manifold whose points correspond to events in space and time. So basically the event of you in your kitchen turning on the coffeemaker this morning is a point of this manifold we could assign spatial and temporal coordinates to.
Similarly our notion of distance between events in this world now incorporates both space and time and is (locally) measured by the so-called metric or fundamental tensor (gμν) which in any given point may be represented by a 4×4 matrix.
Essentially (gμν) together with its first and second derivatives describes the local structure of spacetime in any point, including its Ricci curvature (Rμν).
Conversely, the metric tensor is implicitly determined (up to coordinate transformation) by the so-called mass energy tensor (Tμν) which is kind of a density function characterizing the distribution of mass and energy in the universe.
Specifically the functional dependence between these quantities is given by the famous Einstein equations
Rμν - Λgμν = (8πG/c4)(Tμν-(1/2)Tgμν)
(Where Λ is the cosmological constant, G the gravitational constant, c the speed of light and T the trace of Tμν)
Gravitational Waves
Certain celestial movements may locally disturb the mass energy tensor in a time dependent way, e.g. massive objects such as black holes or neutron stars orbiting each other. By the above Einstein equations, this will also create a small perturbation of our metric tensor g that will start propagating though space - a gravitational wave is born.
By a general theorem from general relativity - Birkhoff's theorem - this can only occur when the mass distribution of the system in question is not spherically symmetric. So a pulsating star or an exploding supernova will in general not generate gravitational waves unless they are for some reason not symmetric.
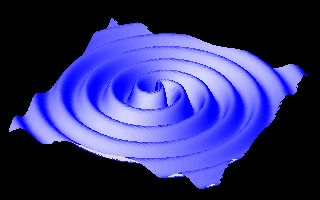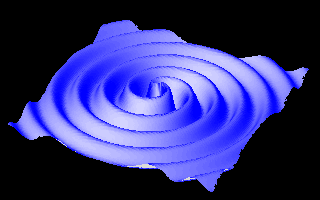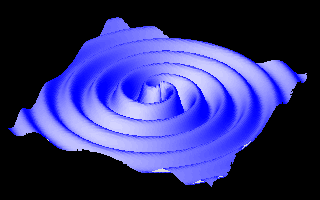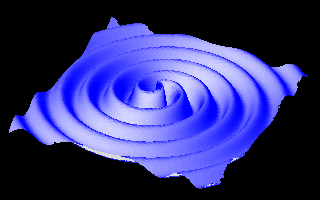
Representation of waves generates by 2 neutron stars orbiting each other, Wikipedia
Gravitational Waves produced by colliding black holes, Simulating eXtreme Spacetimes under CC BY-SA 4.0
Gravitational waves in this context can be considered as ripples in spacetime spreading from their point of origin. These waves propagate through the largely empty universe at the speed of light until they eventually reach the earth - where they alter our spacetime just by a tiny amount.
Depending on the polarization of the gravitational wave they stretch space in some direction and quench it in another. Below you see an exaggerated illustration of a wave on the relative distances of "ring of cosmic dust in a vacuum" in a plane orthogonal to the wave vector:

"Plus" polarization, Wikipedia
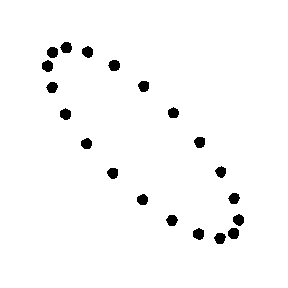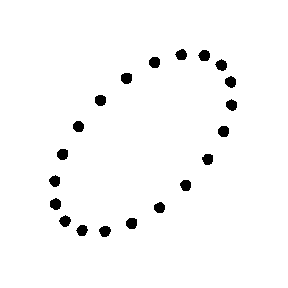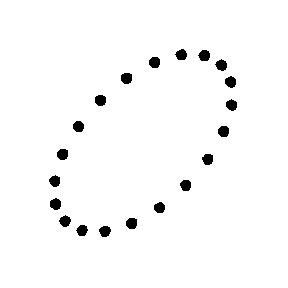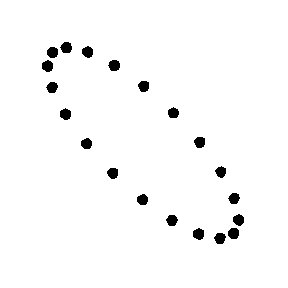
"Cross" polarization, Wikipedia
How do you detect gravitational waves?
This time dependent fluctuation may then be picked up by terrestrial interferometers.
An interferometer consists of two orthogonal long vacuum tubes in in which one coherent laser beam is split up into two half in-phase half-beams by a half-mirror. Each beam travels the length of the tube and is reflected by a mirror at the end. Using another mirror, both beams are then made to converge onto a single optical sensor. This will create an interference pattern of both half-beams that depends on the relative distance both beams travelled (similar to a double slit Laser experiment for instance).
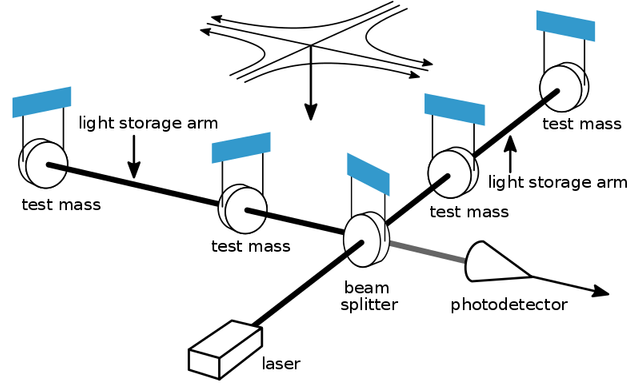
Laser interferometer schematic, Wikipedia
This allows to detect differences (or time dependent fluctuations thereof) in the interferometers "arm length" in order of magnitude of the laser's wavelength, so typically a couple of 100 nanometers. Assuming the overall interferometer dimensions are sufficiently large (state-of-the-art observatories such as the LIGO in the USA or the GEO in Germany have 4km and 600m respectively) this enables astronomers to pick up on really tiny disturbances of the metric tensor by gravitational waves.
In fact those two observartories in addition to several hundred up to thousands of scientists working for or collaborating with them are the major elements to have made the discovery of gravitational waves possible. So I would like to congratulate all of them to their good work and this accomplishment.
This is in essence a brief overview of the topic of gravitational waves and how to to prove their physical existence.
Hopefully this has been enlightening to you and have a nice evening!
References:
- Viatcheslav Mukhanov, Physical Foundations of Cosmology , Cambridge university press
- https://en.wikipedia.org/wiki/Gravitational_wave
- https://www.black-holes.org/
- https://en.wikipedia.org/wiki/Einstein_field_equations
Isn't it interesting that an interferometer like what was developed by Michelson-Morley (1887) to prove/disprove ether was made incredibly larger and used to prove the existence of gravitational waves in 2016 from a theory proposed by Einstein in 1915?
You have a point, that is some serious historic irony ^^
I think it also shows the timescale that scientific advancement takes. It also shows that research and experiments can lead to further discoveries over time with improved technology. I cannot even imagine what the world could be like in another 100 years, but I wonder what experiments are taking place today that will be in the history books of the future
You could have mentioned Virgo too, in the list of examples of observatories :p. The little European I am like to push for that.
Except this small detail, I actually liked reading your post. It is complete and easy to follow. This also strengthen the feeling that I should review GR that I have not used for more than 15 years ... :p
(Note two typos that I found: peudo -> pseudo and observartories -> observatories).
PS: unrelated question: what are you working on? :)
Oh sorry about forgetting that, I shall add it right away and correct the typos :P
I am working on mathematical physics (stuff like spectral theory and mathematical QED) as a PhD student
Oh interesting PhD topic! In which city / group, if I may ask?
I'll tell you next time in the chat :P
I am looking forward to learn it ^^
Nice and good post for geographical scientist and informative for all interested peoples
Geography? :)
Interesting post. The graphics help to understand the information. I wonder when the gravitation wave stretches space, it shortens the distance for a probe to travel.
Really interesting stuff. I had a random question, that I'm not sure makes sense. In the exaggerated illustration of the wave on a ring of dust in a vacuum, if I am one of those specks of dust, I would notice the other speck moving farther away and then closer it seems. Does it have an effect on my clock as well if I'm staring at an atomic clock?
That is a tough question actually.
If I am not completely mistaken gravitational waves do not cause gravitational time dilation in principle (time components in g are unaffected). However they do change the spatial geometry.
But I think the wave would have to have a frequency comparable to atomic transition energies to affect the quantum chemistry of atoms in the atomic clock. I'm not sure.
If you measure time by dividing distance over speed of light then it should affect your measurement if it is quick enough because distances do change
Oh interesting. Yes I was not thinking too specifically about the clock. So it sounds like there's no extra time component to this wave. I guess I was asking since I was imagining that the wave took place on the time-space manifold... Whatever that means.... Weird!
@galotta: watch out that you seem to confuse coordinate time and proper time. The proper time is given by the integration of the line element (defined from the metric). So, a change of the spatial geometry (for one specific observer) does also lead to a time dilatation for that observer. In general, keep in mind that talking about the space component of the geometry or about the time component is observer depedent.
I never mentioned time dilation as an observable effect of gravitational waves though (which is also not the case for a stationary observer ). You are right in that it is observer dependent, I am implicitly referring to first order perturbation theory to minkowski spacetime for a stationary observer on earth which is just the simplest model assumption for the purpose of explaining and detecting gravitational waves.
If we are talking about atomic clocks you would have to solve a Schrödinger equation in explicitly time dependent curved spacetime which I would not want to make an uninformed guess about ^^
And if you measure e.g. the time it takes for light to travel an infinitesimal distance you still measure coordinate time i.e. proper time from the perspective of a stationary observer in only spacially perturbed minkowski space.
@galotta sorry I should have been more specific. I was referring to your comment to @eonwarped "If I am not completely mistaken gravitational waves do not cause gravitational time dilation in principle (time components in g are unaffected)."
Well that is interesting. Why?
Interesting and well-written post, @galotta. I have what might be a silly or ignorant question given my lack of background in physics. You mentioned that gravitational waves travel at the speed of light through the universe. Would it be theoretically possible for us to use something like this for communication in the future over interplanetary distances?
In theory yes, in practice no.
You would need to use ridiculous amounts of energy to produce a tiny signal which you can't even focus properly. The general problem is that gravitation is so much weaker than electromagnetic interaction that you will always want to use light/electromagnetic signals in the first place.
Or you can send hard drives by a reasonably fast ship :D
That makes sense, thanks.
Congratulations @galotta! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOPInteresting how gravity waves travelling at light speed are the result of black holes colliding. This is basically a conversion of matter back to energy, a big bang where all information is erased, and the fixed laws of physics even have a chance to evolve.