The Intricacies of Infinity - Hilbert's Hotel
Hey guys how are you? To motivate today's topic let me ask you a brief question:
Does the set of natural numbers contain more elements than the set of
even natural numbers?
At this point many people might be inclined to respond with.
'Yes, of course! Clearly the former set is a contains both even and odd numbers
so how could it not have more elements?'
But mathematically speaking the answer the answer is:
No, both sets contain the same number of elements, namely countably
infinitely many.
Counting in mathematics
When mathematicians attempt to quantify the number of elements in a given set they talk about cardinality.
One says that 2 given sets have equal cardinality if there exists a bijection between the two, meaning a one to one correspondence between elements of both sets.
In the above example such a bijection is easily contructed by mapping natural number n to even natural number 2n, so 0,1,2,... to 0,2,4,... respectively.
Whenever a set A's cardinality is equal to the cardinality of the natural numbers (ℕ) we describe this set as countably infinite and write |A| = ℵ0.
This last symbol ℵ0 is called Aleph nought and denotes the "lowest order of infinity".
Hilbert's Hotel
A verny nice thought experiment intended to illustrate the intricacies of counting and infinity to his students was devised by David Hilbert (1862-1943), one of the most influential mathematicians of the 20th century:
Suppose you are the manager of a hypothetical hotel with countably infinitely many rooms, for simplicity with consecutive enumeration 0,1,2,... , each with a capacity of one.
Let us (unless otherwise stated) assume that this hotel is already filled to capacity with every room occupied by one guest.
1. A new guest arrives
Now a new guest arrives for his stay in your hotel, how can you accomodate him?
Well technically all of the rooms are booked out and unavailable but since you have infinitely many you can do the following:
Let all of the previous guests move from their respective current room number n to n+1. In other words shift the current guests from rooms 0,1,2,.... to 1,2,3,... . This will leave room 0 empty for the new arrival and everybody else will be accomodated in his new room.
Of course this may be extended analogously to finitely many new arrivals but it gets more interesting:
2. Infinititely many new guests arrive
Suppose a coach with countably infinitely seats and passengers arrives and each of them wants to have a room. What do you do now?
This part is inherently connected to the introductory question we have discussed above. In particular the union of 2 countably sets is also countable infinite, you just have to count smartly.
Clearly our approach from part 1 does not work here, there is no well-defined way of shifting your current guests by infinitely many rooms (to define a map you'd have to specify which guest goes to which room, but there are no rooms with indices "infinity+1", "infinity+2" and so on).
What you can do is "interlacing" current and new guests. Sending each current guest in room n to room 2n will open up all odd numbered rooms 2k+1 (countably infinitely many) for the new arrivals and leave erveryone with a room.
Again, this procedure may be extended to a finite number of coaches with infinite guests arriving. But what if it gets even trickier?
3. Infinitely many coaches arrive
Suppose the hotel is on the shore and a ferry brings new guests. Specifically it carries countably infinitely many coaches with countably infinitely many passengers each - and everyone demands a room!
You might be tempted to repeat our solution from part 2 now. But the iteration of this operation (interlacing old and new guest) is only well-defined for a finite number of repetitions. Plastically speaking, to handle infinite iterations you would once again have to know which map this amounts to and who goes to which room in the end. Otherwise you will just end up interlacing guest for all eternity with no conceivable final state.
But let us agree that you can always create countably infinitely many free rooms by sending n to 2n as above. Without loss of generality one may thus just ignore the old guest for the moment and argue as if the hotel was empty.
One thing which will work is the sending people to different prime number powers:
- Passenger n1 of the 1. coach to room 2n1+1, so passengers 0,1,2,... to rooms 2,4,8,...
- Passenger n2 of the 2. coach to room 3n2+1, so passengers 0,1,2,... to rooms 3,9,27,...
- Passenger n3 of the 3. coach to room 5n3+1, so passengers 0,1,2,... to rooms 5,25,125,...
- ...
This will even leave infinitely many rooms open and because of the uniqueness of prime factorization no room will be assigned more than once.
If you want to assign rooms more economically though you need to us e what is called a pairing function to "enumerate" the ℕ×ℕ new arrivals by ℕ rooms in a 1:1 manner.
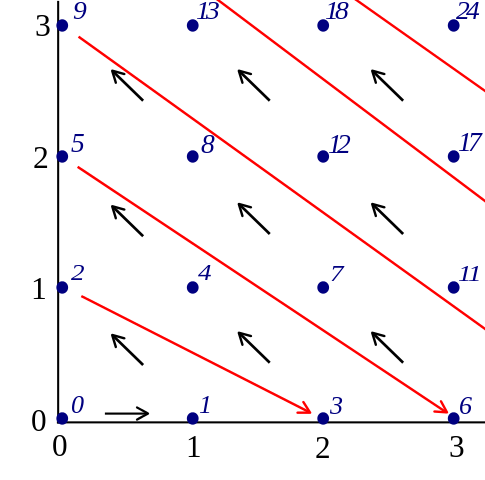
Cronholm144, Pairing natural, CC BY-SA 3.0
As you see in the picture, this can be done by progressing though all pairs of natural numbers "diagonally", e.g.
| (Coach No., Passenger No.) | (0,0) | (1,0) | (0,1) | (2,0) | (1,1) | (0,2) | (3,0) | (2,1) | (1,2) | (0,3) | ... |
|---|---|---|---|---|---|---|---|---|---|---|---|
| Room No. | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | ... |
This corresponds to passenger 0 of coach 0 going to room 0, passenger 0 of coach 1 going to room 1 and so forth.
This procedure working out thereby shows that even the union of countably infinite "copies" of an countably infinite set are still countably infinite.
As it turns out you have to "escalate" step 3 infinitely many times to obtain the prototype of a truly uncountable set:
ℕℕ := ℕ×ℕ×ℕ×ℕ×... is the set of natural number valued sequences and has cardinality |ℕℕ| = ℵ1, which is the "next higher order of infinity" and also corresponds to the cardinality of the real numbers.
As the subscript index suggests it does not stop here, there are ever larger Alephs which represent cardinalities and infinities far beyond the wildest imagination, but maybe this is a story for another time.
I hope that you have learned something new and have a nice day!
References:
Cardinality, Wikipedia
Pairing function, Wikipedia
Hilbert's Hotel, Wikipedia


That is so well put! Super clear! Even someone with a low background in math could follow!
Do you think you would know, anymore, what a person with a low background in math can and cannot follow? ;p
I think anyone can follow anything. It depends on how slowly one explains ^^
Hilberts Hotel looks amazing wow.. nice post .. scientfic I like but nice.. Cheers ;)
Hilbert also came up with my favorite space-filling curve. Fascinating how a one-dimensional object (a line) can fill up at two-dimensional space. Definitely worth checking out!
Yeah, Peano did this first, but Hilbert's solution is leaner I guess ;)
mind blowing, very well put. thanks
No matter how many sets of infinite are attempted to a full capacity, you won't because infinite is meant to be that for a reason, it just won't fill and will infinitely expand because there is no stop to it, just like infinite parallel universes.
Great examples to easily explain this for even those who are not the best or have just woken up like me. :P
math was the worst subject when I was attempting to school, now its seems very interesting :P
Great! Thank you, I really love mathematics :)
Congratulations @galotta! You have completed some achievement on Steemit and have been rewarded with new badge(s) :
Click on any badge to view your own Board of Honor on SteemitBoard.
For more information about SteemitBoard, click here
If you no longer want to receive notifications, reply to this comment with the word
STOP@galotta, it is very nice and nice very complex explained. you have a good math qualification. I am amazed to you
hi....follow me and i follow u sure
I wrote about this subject a few days ago, in a post in Spanish.