A mathematical annecdote that will BLOW your mind!
A well-known anecdote of the mathematician David Hilbert (1881-1966), who was a follower of Cantor (which was not at all mathematicians at that time!), Is an excellent example of the concept of "countable infinite".

A normal hotel can be fully occupied, so that a just arrived guest finds all rooms occupied. This is a problem that does not apply to Hilbert's hotel. Hilbert's hotel has countless hotel rooms. Each of the hotel rooms is numbered, so there are rooms 1, 2, 3, 4, 5, 6, 7, 8, 9, ... .. and so on. Now, a new guest arrives at the front desk asking for a room, but all the rooms are occupied.
An exception in any other hotel, but not in this hotel.

All guests are asked to change the room and move on to a room, which means the current number of rooms, For example the number n +1. The rooms are very clean and the staff is very friendly.Now our new guest can move into the free room 1.

If 100 new guests arrive, this would be just as little a problem as we would leave the guests in the occupied rooms instead of a number around 100, and the problem would be solved.

But now the scenario that the usual imaginative ability of infinity breaks:
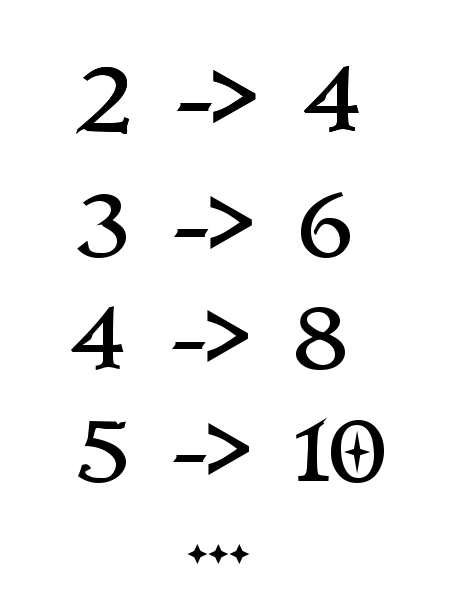
An extraordinary train with infinitely many passengers stops in front of the hotel. Infinitely many guests looking for a room in Hilbert's fully booked hotel. Again, this is no problem. The new rooms number of guests who have already been consulted can be determined by taking the current room number n x 2, or by adding them together. For the room numbers 1, the new guests receive the new number 2, for room 2 the new number 4, for 3 the new number 6 ... to infinity, and look for their new room on the endless corridor while the now vacated rooms, All of which have odd room numbers, can be occupied by the countless countless new guests.

The concept of infinity is a fascinating one, albeit a hard one to grasp. There are several intertwined mathematical notions of infinity, each with its practical usefulness and axiomatic framework. The set theoretical notion which allows a distinction between countably and uncountably infinite sets is a remarkable idea which pushes the boundaries of logic. Hilbert's Grand Hotel is a clever way to convey the subtleties of a countable infinity in everyday's terms. It would be nice to develop the analogy further in order to explain the difference between a countable and an uncountable infinity.
Than you for the probs!
I already wrote something about the difference of countable and uncountable infinity and you're motivating me to post it on steemit :)
You should definitely post it!
Thanks, nice explanation. And I agree with some other comments that the next mind-blowing consequence is the distinction between countable and uncountable infinities.
!-=o0o=-!
To follow curated math content follow @math-trail.
If you wish @math-trail to follow you then read this article.
I guess we shouldn't be too concerned about the amount of time it takes.
If we can do everything at very near the speed of light it won't take long at all.
That is except to a stationary observer that would have aged and died long before everyone checked in, or is it the other way around?
Infinities are indeed very important quantities. Dealing with infinities is actually one of the tasks I am handling almost on an everyday basis with my particle physics work :)
Interesting. I work in particle physics as well, and even if it is often not in a completely explicit fashion, infinities do tend to pop up everywhere and need to be properly take care of. I'm sure you know what I mean. What have you been working on lately?
I work on many things at the momentm, all connected to theoretical particle physics: LHC, beyond the standard model physics, dark matter, future experiments, precision calculations, etc... On too many things I may want to say ^^
What are you working on?
EDIT: just noticed you answered below ;)
Ok that REALLY sounds mind-blowing!
Im doing an apprenticeship, it does not have anything to do with science. I've just been interested in such things and read about them in my spare time :)
It is indeed cool . I like what I do, and I try to share this love for science with others :)
PS: Note that I am a physicist and not a physician (cf. your other message)
I like the linking to the world of physics!
But I am really not a physician, nonetheless I love this anecdote!
What are you doin' in your everyday basis?
Well, the specific tasks vary a bit, but I'm studying the dynamics of strong interactions using an effective model. Our aim right now is to be able to fit our model to describe some of the phenomenology of the light mesons and then to do some thermodynamic analysis. I'd gladly elaborate on this, but I'm not sure this is the proper place to do so. Thank you for asking, though. Is your work related with science as well?
Please do elaborate and try to discuss what you are working on. I would be more than happy to read!
I tried to do that in the past, and some Steemians really liked it. By the way, now I got enough info and I found who you were. I hope we will meet one day ^^
PS: as soon as the steemit chat is back, you may want to visit us on the #steemSTEM channel.